√70以上 tan^2x 1=sec^2x proof 529650-Tan^2x+1=sec^2x proof

Tan^2xtan^2y=sec^2xsec^2y and, how do you factor and simplify, cscx(sin^2xcos^2xtanx)/sinxcosx calculus identities prove this identity sec(y) cos(y)= tan(y)sin(y) THANK YOU!!Specifically, cosecx=1/sinx tanx=sinx/cosx 1/cosx=secx and possibly tan^2x 1= sec^2x I began by changing cosecx into 1/sinx in hopes of simplifying the fraction Tan^2x(1/sinx)/sinx I then simplified the fraction by multiplying the reciprocal of the top fraction (sinx/1) by the numerator and the denominator This gave me tan^2x/sin^2x I then substituted tan^2x in the numerator for theProving Trigonometric Identities Calculator online with solution and steps Detailed step by step solutions to your Proving Trigonometric Identities problems online with our math solver and calculator Solved exercises of Proving Trigonometric Identities

Y Log Squareroot Of 1 Tanx 1 Tanx Then Prove That Dy Dx Sec2x Mathematics Topperlearning Com Vben5mcc

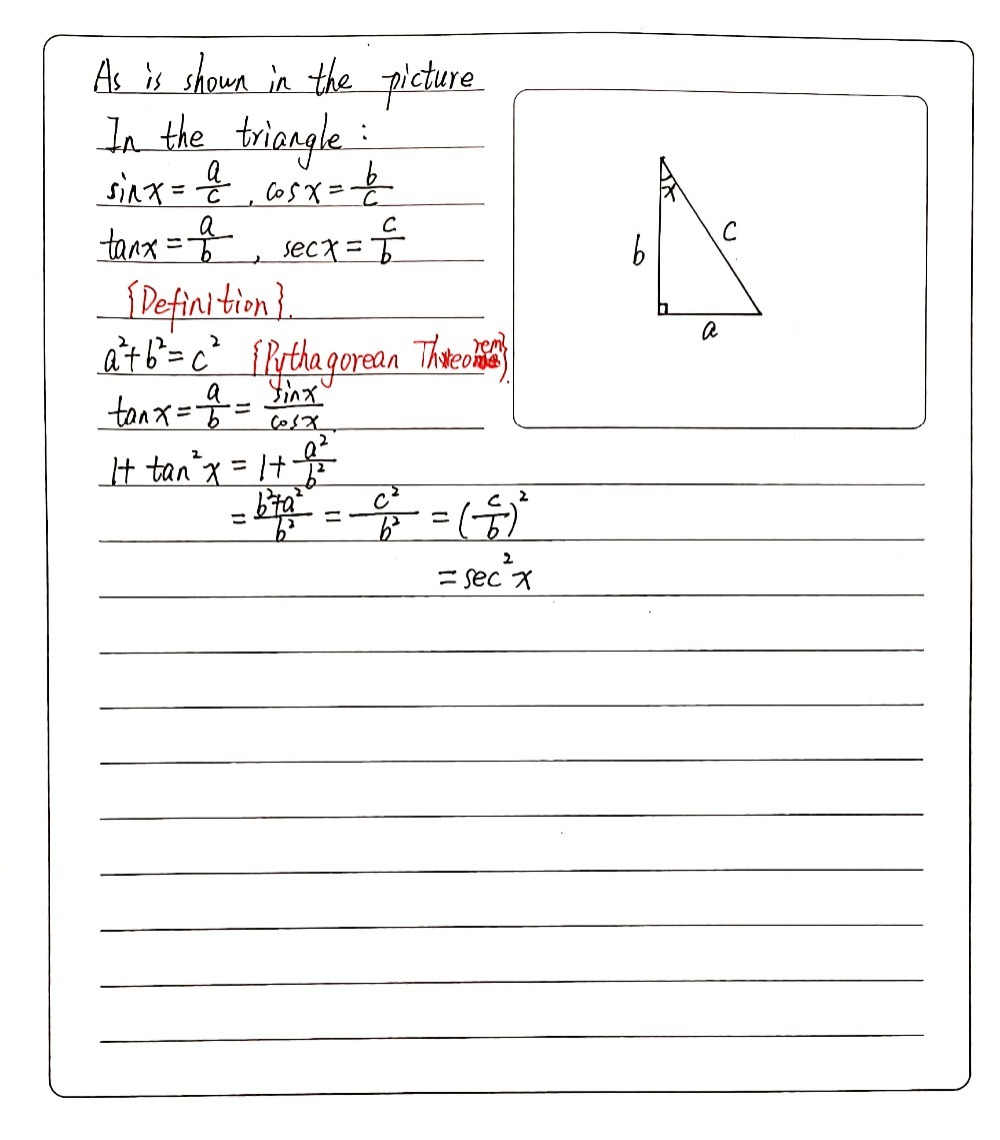
Tan^2x+1=sec^2x proof

Tan^2x+1=sec^2x proof- · tan^2 x1=sec^2x So to get 1 on the other side of the equal sign wouldn't it be sec^2xtan^2x=1?Verify (1tan^2x)/(1cot^2x) = 1sec^2x Answered by a verified Math Tutor or Teacher We use cookies to give you the best possible experience on our website By continuing to use this site you consent to the use of cookies on your device as described in

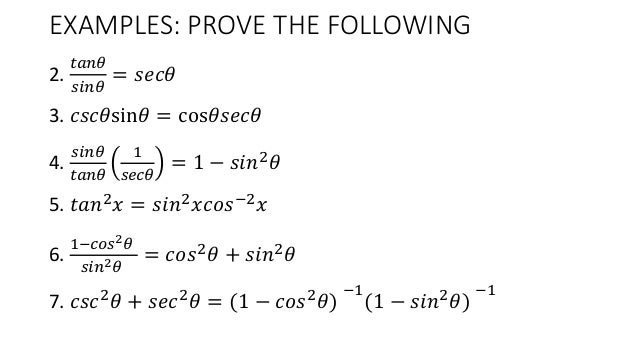
Trigonometry Trigonometric Identities

 · You should be able to get each side down to "cos2(x) / 1 cos(x)" You can then do the proof by working down one side to this point, and then working backwards up the other side to get to that side's starting point Eliz C ChrisRR New member Joined Oct 28, 06 Messages 2 Oct 28, 06 #3 yes thats the problem not sure what u meant my professor told usAbout Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How works Test new features Press Copyright Contact us CreatorsFree math lessons and math homework help from basic math to algebra, geometry and beyond Students, teachers, parents, and everyone can find solutions to their math problems instantly

Proof of the Pythagorean trig identity This is the currently selected item Using the Pythagorean trig identity Practice Use the Pythagorean identity Pythagorean identity review Next lesson Trigonometric values of special angles Video transcript so let's review the unit circle definition of trig functions a little bit right over here I've drawn a unit circle and when we say unit circleHi, I'm struggling with 2 questions asking to prove trig identities 1) Prove sec 2 x (x) cosec 2 x= 2 cot 2 x tan 2 x I've started on the right hand side and wrote 2sin 2 x 2cos 2 x (cos 2 x)(/sin 2 x) (sin 2 x)/(cos 2 x), and then split up these fractions to get 3/sin^2x 3/cos^x = 3cosec^2x 3sec^2x = cosec^2x sec^2x (however, the proof says x not ), what have I done wrong?Solve for x tan(2x)=1 Take the inverse tangent of both sides of the equation to extract from inside the tangent The exact value of is Divide each term by and simplify Tap for more steps Divide each term in by Cancel the common factor of Tap for more steps Cancel the common factor Divide by Multiply Tap for more steps Multiply and Multiply by The tangent function is

While what I've done so far is not a proof, I have managed to get the two sides to meet in the middle And sometimes that seems to be the only way to do a proof work on the two sides until they meet in the middle, and then write something that looks like magic I'm going to start with the LHS, work down to where the two sides meet, and then work up the RHS until I get back to the · In mathematics, the trigonometric functions (also called circular functions, angle functions or goniometric functions) are real functions which relate an angle of a rightangled triangle to ratios of two side lengths They are widely used in all sciences that are related to geometry, such as navigation, solid mechanics, celestial mechanics, geodesy, and many othersFree math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics homework questions with stepbystep explanations, just like a math tutor

Prove The Identity 1 Sec 2 X Cos 2 X 1 Cosec 2 X Sin 2 X Sin 2 X Cos 2 X 1 Sin 2 X Cos 2 X 2 Sin 2 X Cos 2 X Sarthaks Econnect Largest Online Education Community

Prove That Sec 4x 1 Sin 4x 2tan 2x 1 Maths Meritnation Com

 · Ex 34, 8 Find the general solution of the equation sec2 2x = 1 – tan 2x sec2 2x = 1 – tan 2x 1 tan2 2x = 1 – tan2x tan2 2x tan2x = 1 – 1 tan2 2x tan2x = 0 tan 2x (tan2x 1) = 0 Hence We know that sec2 x = 1 tan2 x So, sec2 2x = 1 tan2 2x tan 2x = 0 ta · (1/tan^2x)(tan^2x/tan^2x)= sec^2x1= csc^2x=csc^2x Approved by eNotes Editorial Team We'll help your grades soar Start your 48hourTrigonometric Identities and Formulas Below are some of the most important definitions, identities and formulas in trigonometry Trigonometric Functions of Acute Angles

Consider The Following Equations 1 Cosec 2x Sec 2x Cosec 2xsec 2x 2 Sec 2x Tan 2x Sec 2xtan 2x 3 Cosec 2x Tan 2x Cot 2x

What Is Sec 2x Equal To

Algebra > Trigonometrybasics> SOLUTION verify the idenity sec^4x – sec^2x = tan^4x tan^2x'' Log On Algebra Trigonometry Section Solvers Solvers Lessons Lessons Answers archive Answers Click here to see ALL problems on Trigonometrybasics;I'm very confused or could you change it into sin^2x/ cos^2x 1/cos^2x=1 which would be sin^2x/cos^2x=1 which would be tan^2x=1 SO confused!Trigonometric Identities In this unit we are going to look at trigonometric identities and how to use them to solve trigonometricequations

Is There Any Other Way To Establish This Trig Identity Frac Sec X 1 Tan X Frac Sin X 1 Cos X Mathematics Stack Exchange

Integral Of Tan 3x Sec 2x

Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more(sin x cos x) ^2 a 12sinxcosx b sec^2x−tan^2x2cosxsinx csec x 2 sin x/sec x d sin^2xcos^2x e 12cos (pi / 2 Trig Identities Prove the following identities 13 tan(x) sec(x) = (cos(x)) / (1sin(x)) *Sorry for any confusing parenthesis* My work I simplified the left side to a ((sinx) / (cosx)) (1 / cosx) , then b (sinx 1) / cosx = (cos(x)) / (1sin(x)) I don't knowProving $(\sec^2x\tan^2x)(\csc^2x\cot^2x)=12\sec^2x\csc^2x$ and $\frac{\cos x}{1\tan x}\frac{\sin x}{1\cot x} = \sin x \cos x $

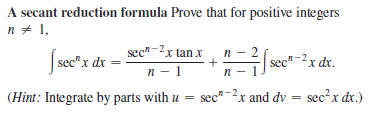
Answered A Secant Reduction Formula Prove That Bartleby

Verifying A Trigonometric Identity Cos X 1 Tan 2 X Cos X Sec X 1 Youtube

D is the differential operator, int is the integration operator, C is the constant of integration Identities tan x = sin x/cos x equation 1 cot x = cos x/sin x equation 2 sec x = 1/cos x equation 3 csc x = 1/sin x equation 4Follow Report by Rahulagrawal2 Log in to add a comment2 x = sec ⁡ 2 x I started this by making sec 1/cos and using the double angle identity for that and it didn't work at all in any way ever Not sure why I can't do that, but something was wrong Anyways I looked at the solutions manual and they magic out 1 tan ⁡ x tan

Analytic Trig Ppt Video Online Download

F 2tanx 1 Tan 2x Cos2x 1 Sec 2x 2tanx 2

Purplemath In mathematics, an "identity" is an equation which is always true These can be "trivially" true, like "x = x" or usefully true, such as the Pythagorean Theorem's "a 2 b 2 = c 2" for right trianglesThere are loads of trigonometric identities, but the following are the ones you're most likely to see and useMath\int \frac{1\tan^2x}{1\tan^2x} \,dx/math math\int \frac{1\tan^2x}{\sec^2x} \,dx/math math\int \frac{1\tan^2x}{\frac{1}{\cos^2x}} \,dx/math math · See the proof below We need tanx=sinx/cosx sin^2xcos^2x=1 secx=1/cosx Therefore, LHS=tan^2x1 =sin^2x/cos^2x1 =(sin^2xcos^2x)/cos^2x =1/cos^2x =sec^2x =RHS QED

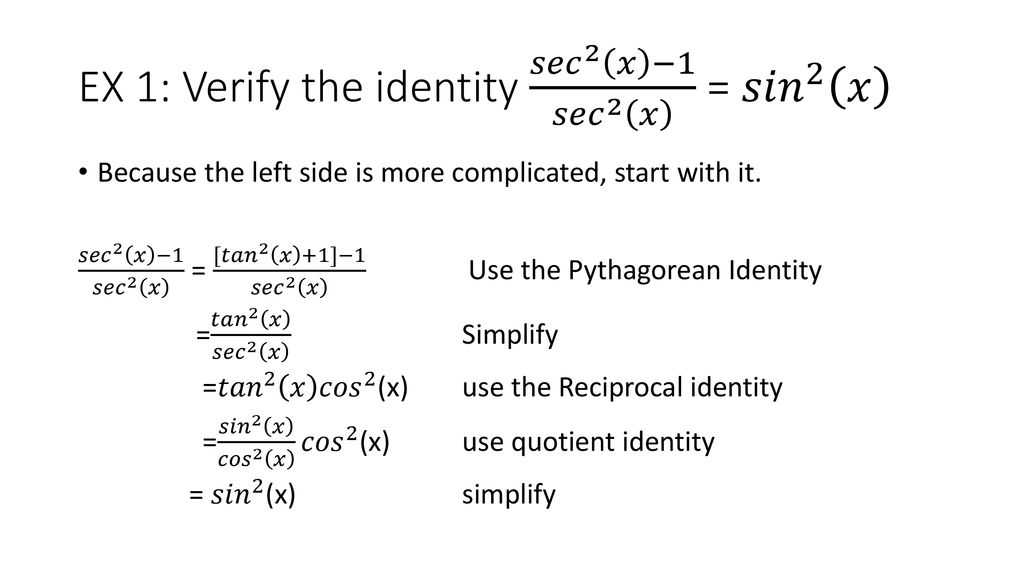
Ch Ppt Download

Solved Verify The Identity 4 2 Tan X Secx2tan X 1 Whic Chegg Com

 · Set your main menu in Appearance > Menus Search 4142 Frederick ST, Birmingham B1 3HNProof 1 − cos ⁡ θ θ = 1 − cos 2 ⁡ θ θ ( 1 cos ⁡ θ ) = sin 2 ⁡ θ θ ( 1 cos ⁡ θ ) = ( sin ⁡ θ θ ) × sin ⁡ θ × ( 1 1 cos ⁡ θ ) {\displaystyle {\begin{aligned}{\frac {1\cos \theta }{\theta }}&={\frac {1\cos ^{2}\theta }{\theta (1\cos \theta )}}\\&={\frac {\sin ^{2}\theta }{\theta (1\cos \theta )}}\\&=\left({\frac {\sin \theta }{\theta }}\right)\times \sin \theta \times \left({\frac {1}{1\cos \theta }}\right)\\\end{aligned}}}The trigonometric identity `(tan^2x)/(1tan^2x) = sin^2x` has to be proved Start with the left hand side `(tan^2x)/(1tan^2x)` Substitute `tanx = sin x/cos x`

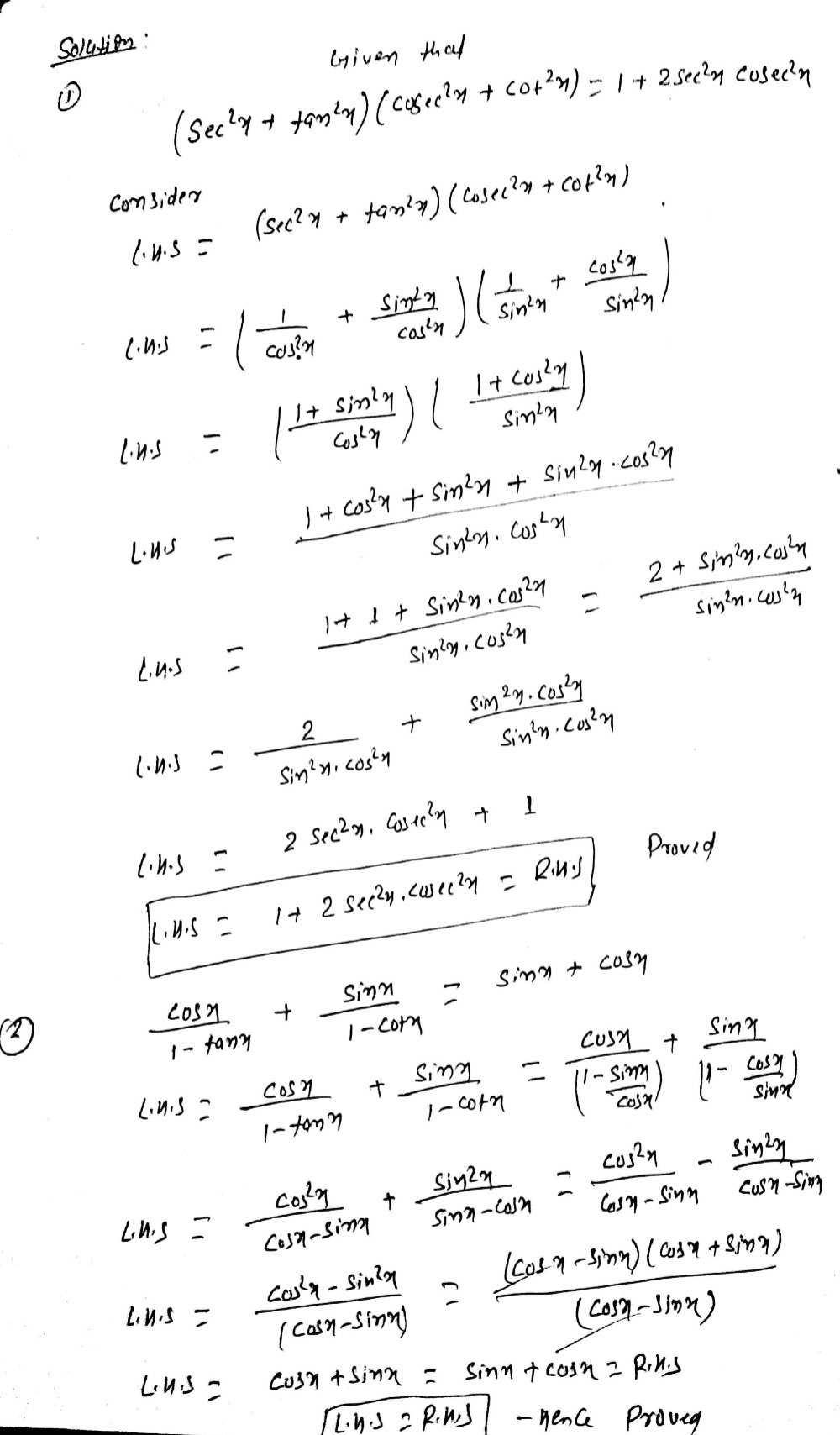
Prove The Following Identities I Sec 2x Tan 2xcos Gauthmath

Rd Sharma Solutions For Class 11 Chapter 5 Trigonometric Functions Download Free Pdf

 · Proof This time we start with the cosine of the sum of two angles cos α β) = cos α cos β − sin α sin β, and once again replace β with α on both the LHS and RHS, as follows LHS = cos(α α) = cos(2α) RHS = cos α cos α − sin α sin α = cos 2 α − sin 2 α Different forms of the Cosine Double Angle Result By using the result sin 2 α cos 2 α = 1, (which we found in TD Follow • 3 Add comment More Report 2 Answers By Expert Tutors BestLegend x and y are independent variables, ;

1 Tan 2x 1 Tan 2 X 1 2cos 2 X Youtube

1 Cosx 1 Cosx Tan 2x Secx 1 2prove Brainly In

Question verify the idenity sec^4x – sec^2x = tan^4x tan^2x'' Answer by Edwin McCravy() (Show Source) You can · Find an answer to your question tan^2x 1 = sec^2x PROVE hopelafave hopelafave 1402 Math Secondary School answered Tan^2x 1 = sec^2x PROVE 1 See answer hopelafave is waiting for your help Add your answer and earn pointsTrigonometry is the study of the relationship between the sides and angles of a triangle We know that i) sin² θ cos² θ = 1 , ii) 1 tan² θ = sec² θ , iii) cot² θ 1 = cosec² θ SOLUTION Given sec⁴ θ – sec²θ = tan⁴θ tan²θ LHS = sec⁴ θ

Egua8pk Nue Zm

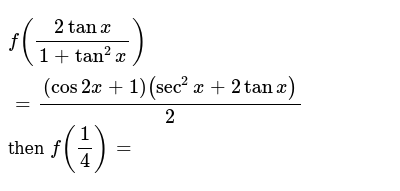
F 2tanx 1 Tan 2x Cos2x 1 Sec 2x 2tanx 2 Then F 1 4

Free trigonometric identities list trigonometric identities by request stepbystepProof Suppose x is any real number such that XX > 2 1 / 2 (*) Multiply both sides of the inequality by 2 to obtain 2x – 2x > 1 or, equivalently, Select Select Now by definition of floor, x < x 1, and hence Select (**) Select 2x 22x Put inequalities (*) and (**) together to obtain 2L Select XFree integral calculator solve indefinite, definite and multiple integrals with all the steps Type in any integral to get the solution, steps and graph

Sec 6x Tan 6x 1 2 Tan 2x Sec 2x Important Difficult Trigonometric Identity Youtube

2sinxcosx2 Cos X Answer Gamers Smart

SOLUTION prove that tanx(cotxtanx)=sec^2x i have no idea how to do this please help thank youSolve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more · The Second Derivative Of sec^2x To calculate the second derivative of a function, differentiate the first derivative From above, we found that the first derivative of sec^2x = 2sec 2 (x)tan(x) So to find the second derivative of sec^2x, we need to differentiate 2sec 2 (x)tan(x) We can use the product and chain rules, and then simplify to find the derivative of 2sec 2 (x)tan(x) is

Ex 3 3 23 Prove Tan 4x 4 Tan X 1 Tan2 X 1 6tan2x

Prove The Following Sec6x Tan6x 1 3sec2x Tan2x Maths Introduction To Trigonometry Meritnation Com

 · \(1\tan^2x = \sec^2x\) (the Pythagorean Theorem) If the integrand can be manipulated to separate a \(\sec^2x\) term with the remaining secant power even, or if a \(\sec x\tan x\) term can be separated with the remaining \(\tan x\) power even, the Pythagorean Theorem can be employed, leading to a simple substitution This strategy is outlined · 资料 cotx乘tan2x=sec2x tan2x=2tanx/ （ 4102 1tanx的平方） 1653 设tanx=t,则原方程 变为 ： 2t/（1t^2）1/t=0 即：2t^2 (1t^2)=0 tProve that cot x tan 2x1 =sec 2x math Prove that the equation Is an identity Sec^4x Tan^4x = Sec^2x Tan^2x Trigonometry How do you verify the equation is an identity?

How To Show That Math Tan 2 X Sec 2 X 1 Math Quora

7 Proving Ids Trig Functions Identities

 · Tan ^2xsec^2x=3 solve this problem Ask for details ;

Trig Identity Sec2x Minus Tan2x T10 Youtube

Every Day I M Calculatin I D3 Unit Q Pythagorean Identities

Prove That Tanh2 X Sech2x 1 Stumbling Robot

Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo

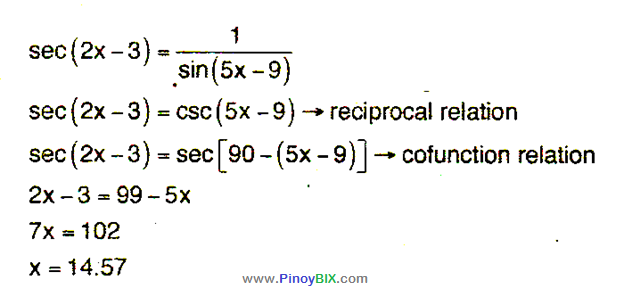
Solution If Sec 2x 3 1 Sin 5x 9 Determine X In Degrees

If Cosx 1 10 Then Prove That Sec 2x Tan 2x 1 Trigonometric Value Sci Pi

Prove That Tan 2 2x Tan 2 X1 Tan 2 2x Tan 2 X Tan 3x Tan X

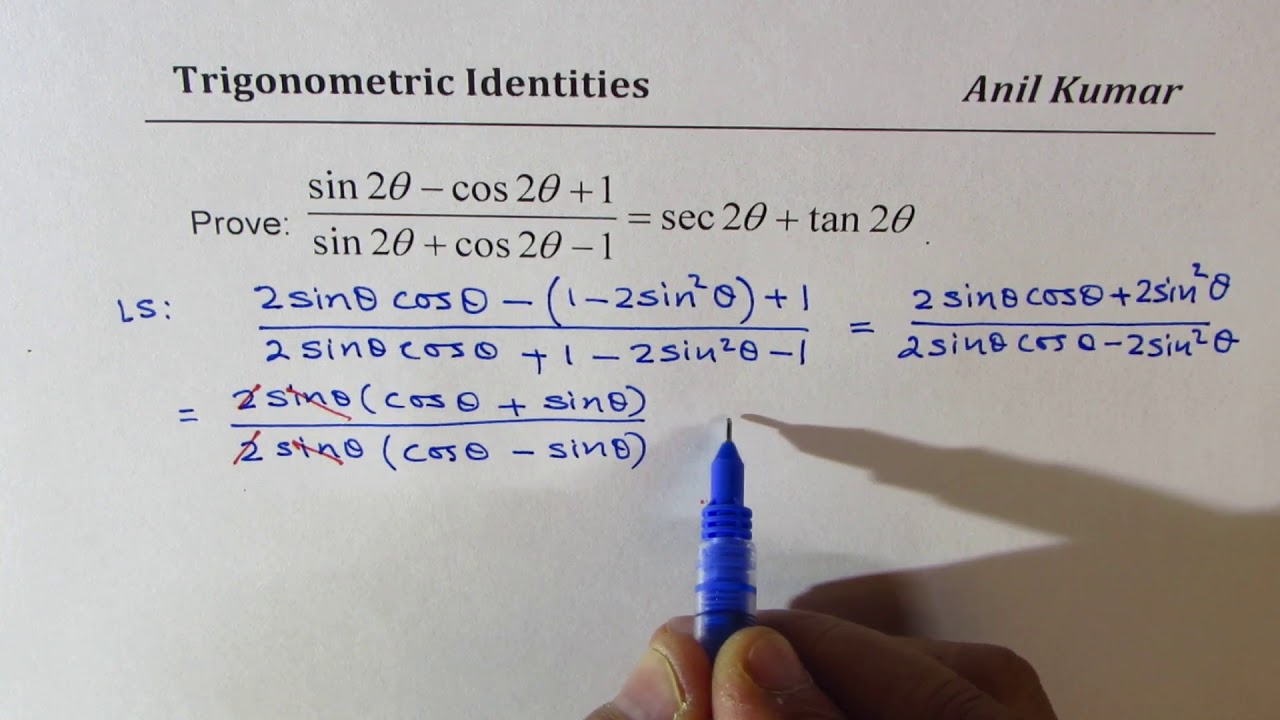
Trigonometric Identity In Double Angle Sin2x Cos2x 1 Sin2x Cos2x 1 Sec2x Tan2x Youtube

If Sec X Tan X A Then A 2 1a 2 1

Y Log Squareroot Of 1 Tanx 1 Tanx Then Prove That Dy Dx Sec2x Mathematics Topperlearning Com Vben5mcc

If Sec A X 1 4x Then Prove That Sec A Tan A 2x Or 1 2x Brainly In

Prove That 1 Sec 2theta 1 Sec 4theta 1 Sec 8theta 1 Sec 2 Ntheta Tan 2 Ntheta Cottheta

Derivative Of Tan 2x Sec 2x

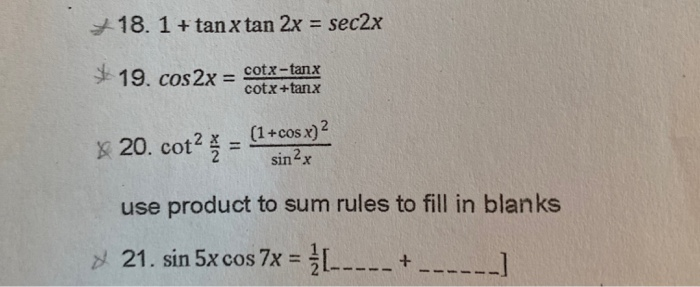
Solved 18 1 Tan X Tan 2x Sec2x 19 Cos2x Cotx T Chegg Com

How Many Can You Derive From First Principles Ppt Download

Solved Prove The Following Trig Identity Sec 2 X 2secx Cosx Cos 2 X Tan 2 X Sin 2 X Course Hero

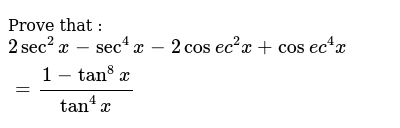
Prove That 2sec 2x Sec 4x 2cosec 2x Cosec 4x 1 Tan 8x Tan 4x

Prove That Sec 4x Sec 2x Tan 2 X Tan 4x

Omtex Classes Iv Sec6x Tan6x 1 3 Sec2x Tan2x

Trigonometry Trigonometric Identities

Find The General Solution For The Equation Sec 2 2x 1 Tan 2x Sarthaks Econnect Largest Online Education Community

If Sec Tita X 1 4x Sec Tita Tan Tita 2x Or 1 2x Mathematics Topperlearning Com 15o2fj

Xswjl Vrk4qd7m

How Does One Verify Cos 2x Sin 2x 1 Tan 2x Cos 2x Socratic

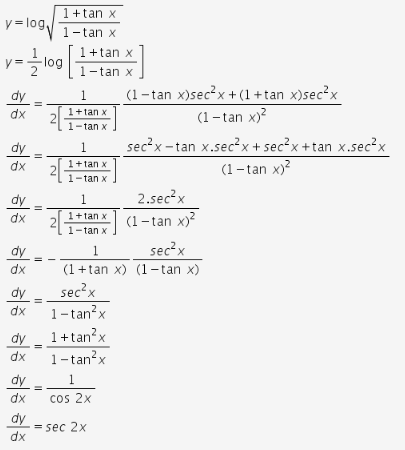
Y Log Squareroot Of 1 Tanx 1 Tanx Then Prove That Dy Dx Sec2x Cbse Class 12 Learn Cbse Forum

Solved 2 Prove The Following Trig Identities A Prove Chegg Com

Integrate Sec 2x Method 2

Integrate Sec 2x Method 1

Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo

If Y Tan 1 X 2 Prove That 1 X2 2y2 2x 1 X2 Y1 2 Explain In Great Detail Mathematics Topperlearning Com Z68xug

Prove The Identity Tan P 4 X Tan P 4 X 2 Sec 2x Sarthaks Econnect Largest Online Education Community

Solved For 1 Through 4 Prove The Following Identities 1 Chegg Com

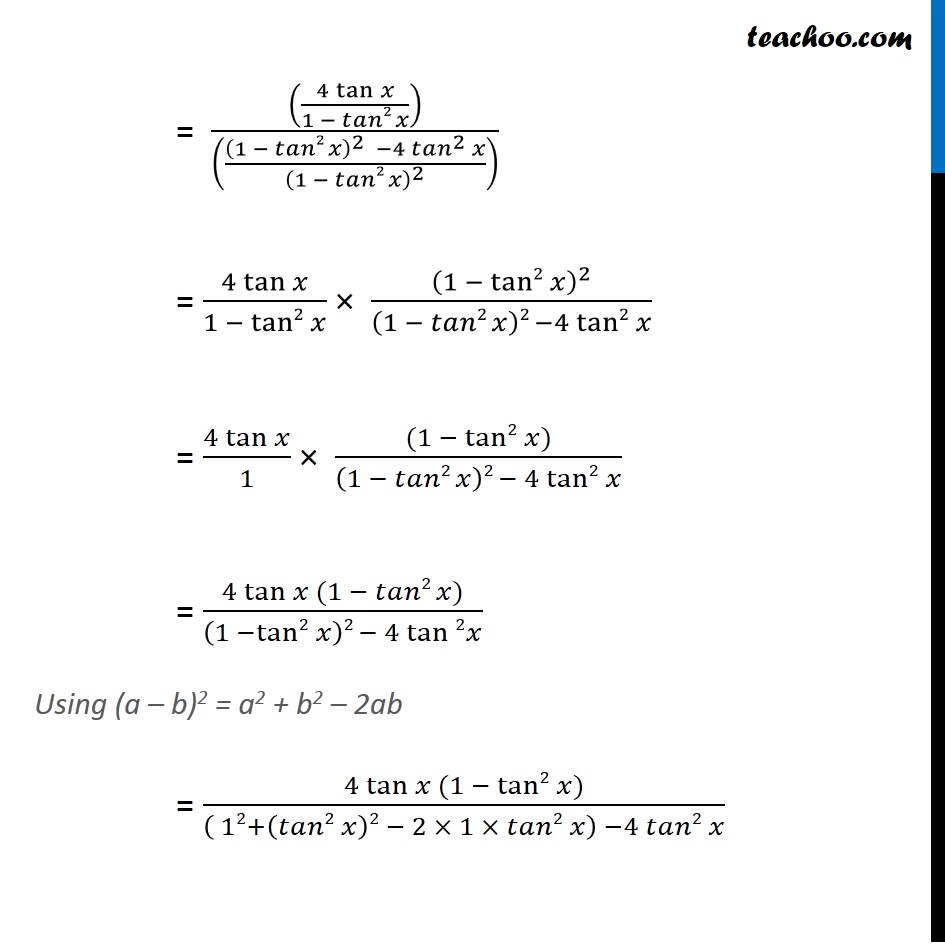
Ex 3 3 23 Prove Tan 4x 4 Tan X 1 Tan2 X 1 6tan2x

How To Prove Csc 2x 1 Tan 2x 1 Is Equivalent To Cosec 2x Quora

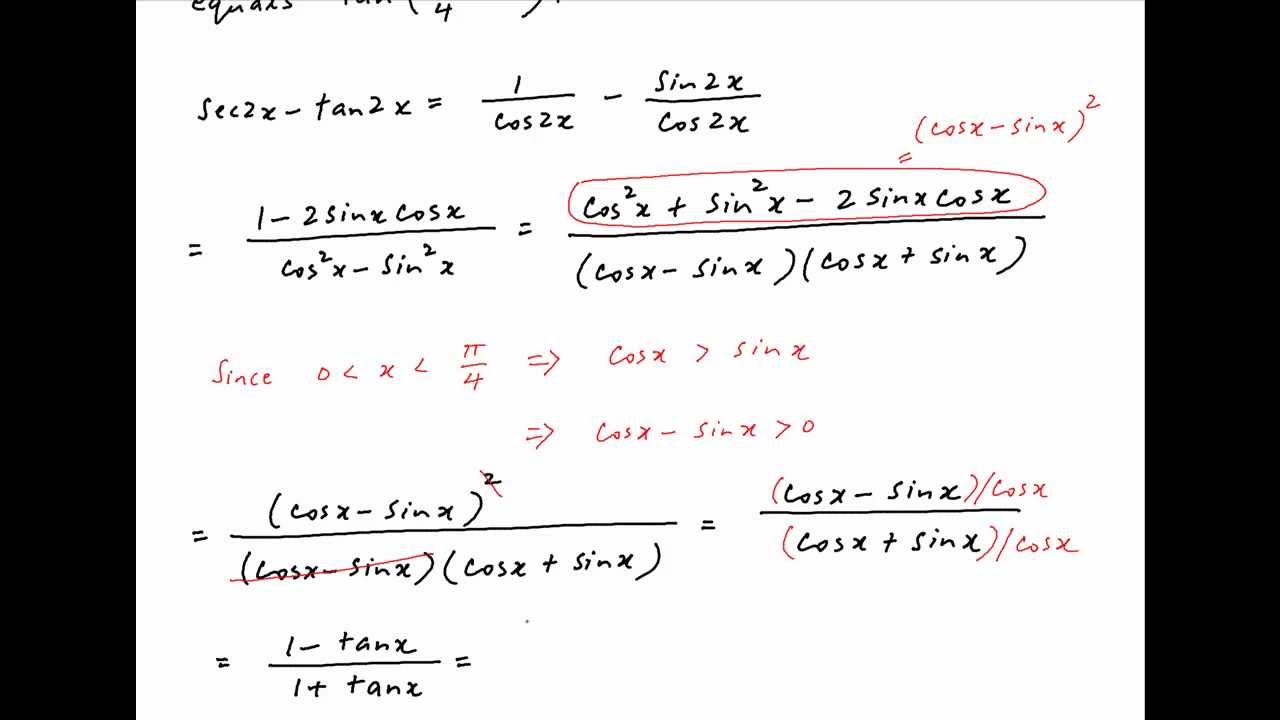
Iit 1994 Prove That Sec2x Tan2x Tan Pi 4 X When X Lies Between 0 And Pi 4 Youtube

Solved 2 Prove The Following Trig Identities A Prove Chegg Com

Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo

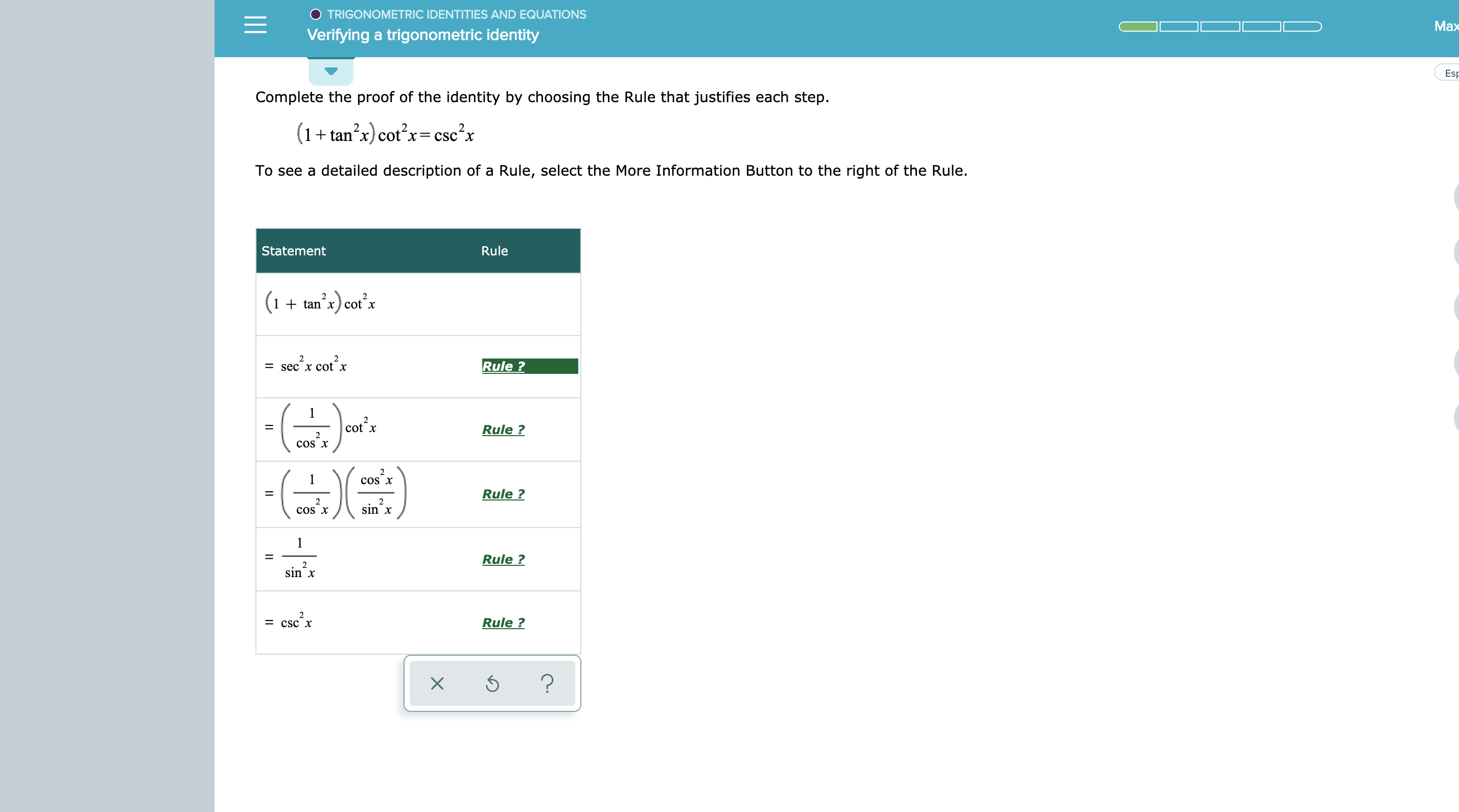
Answered Trigonometric Identities And Equations Bartleby

If Sec 8 X 1 4x Prove That Sec 8 Tan 8 2x Or 1 2x Sarthaks Econnect Largest Online Education Community

How To Show That Math Tan 2 X Sec 2 X 1 Math Quora

How Do You Simplify 1 Tan 2 X 1 Tan 2 X Socratic

Proof Tan2 1 Sec2 Cute766

Questions And Answers Cbse Icse Solutions Cbse Icse Study Materials Online Learning Question Papers Classnotes Practice Test Mcq

1 Tan 2x 1 Cos 2x Sin 2x 2sin 4x 1 Sin 2x Trigonometric Identities Mcr3u Youtube

Solved Consider The Possible Identity Tan 2x Cos 2x 1 Cos 2x Sec 2x A State Any Non Permissible Values B Attempt To Verify Possible Identity Course Hero

Maths Xii Pracice Qns For Chapter 1 And 2

What Would Be Steps In Proving That Tan2x Secx 1 1 Sec X Socratic

How Do You Integrate Int Sec 2x 1 Tanx 3 Using Cute766

Prove The Following Identities I 4 Marks Each A Gauthmath

Solved 1 Given The Triangle Below Calculate The Followi Chegg Com

How Do You Prove The Identities Cosx Secx Sinx Cscx Sec 2x Tan 2x Socratic

C3 Chapters 6 7 Trigonometry Ppt Download

Sec 2 X Cosec 2x Tan X Cot X Prove It Brainly In

If Sec A X 14x Then Prove That Sec A Tan A 2x Or 12x Maths Introduction To Trigonometry Meritnation Com

Math Project By Orchid Hassan

Proof Tan 2 1 Sec 2 Youtube

If Sec Theta X 1 4x Then Prove That Tan Theta Sec Theta 2x Or 1 2x Brainly In

Sec 8x 1 Sec 4x 1 Tan 8x Tan 2x Maths Trigonometric Functions Meritnation Com

How To Prove That 2tanx Sec 2 X Sin 2x Quora

Tan2x ただの悪魔の画像

How Do You Verify 4tan 4 Tan 2x 3 Sec 2x 4tan 2x 3 Kinda Hard Please With All The Steps Thanks Socratic

Prove 1 Tan 2 Theta Sec 2 Theta Mathematics Stack Exchange

How Do You Simplify Sec 4x Tan 4x Sec 2x Tan 2x Socratic

Prove The Following I Sec 6 X Tan 6 X 1 3 Sec 2 X Tan 2 X Ii Tan8 Sec8 1 Sec8 1 Tan8 Sarthaks Econnect Largest Online Education Community

How To Prove That Math Frac Sec 8x 1 Sec 4x 1 Frac Tan 8x Tan 2x Math Quora

How Do You Prove Tan 2x Secx 1 1 Secx Socratic

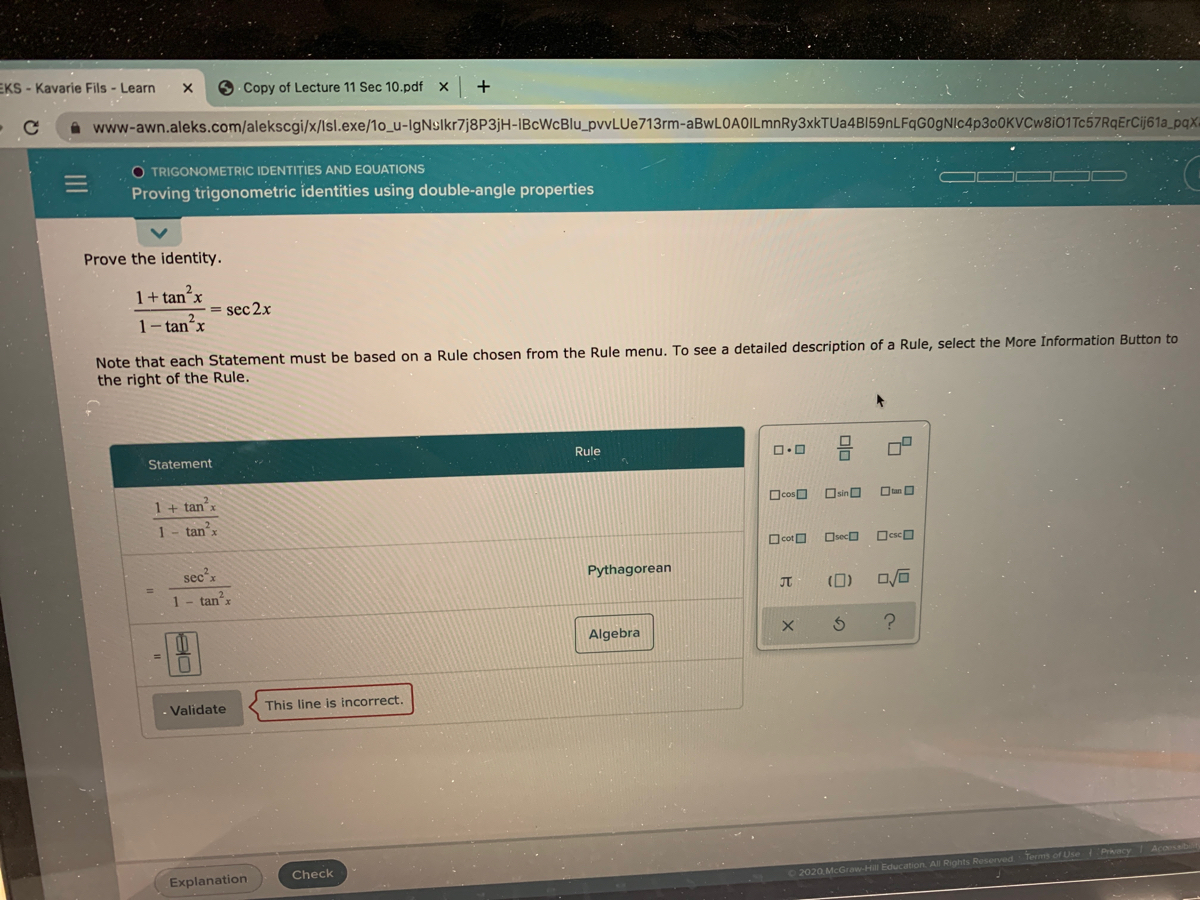
Answered Prove The Identity 1 Tanx Sec 2x 2 1 Bartleby

If Sec 2x Tanx Tan 2x Tan 2x Sec 2x Tanx Tanx Tan

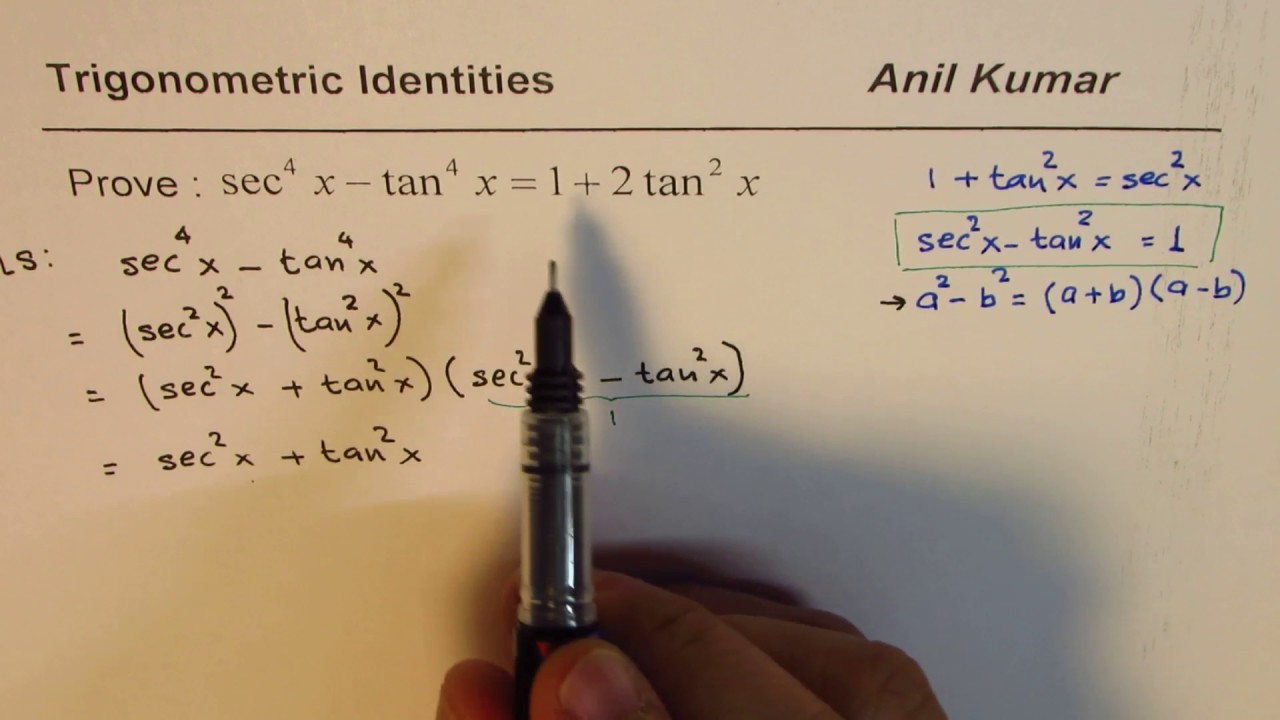
Trig Identity Sec 4x Tan 4x 1 2tan 2x Youtube

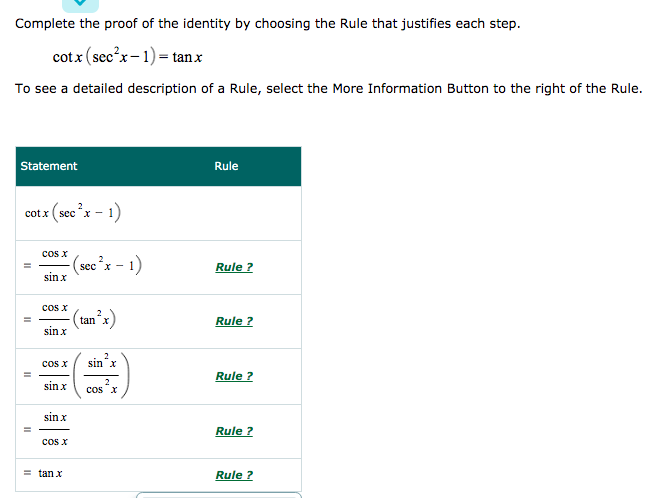
Solved Complete The Proof Of The Identity By Choosing The Chegg Com

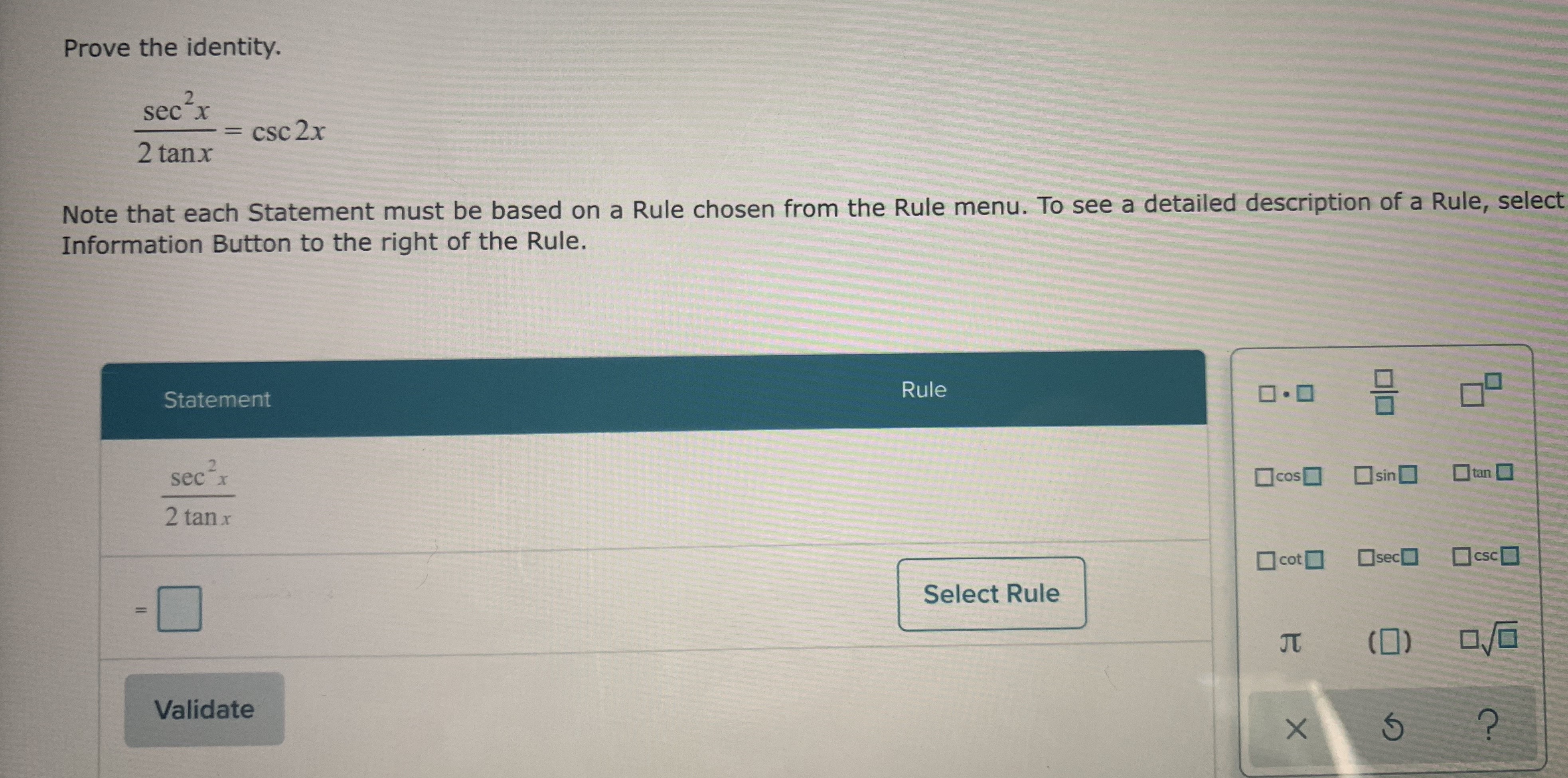
Answered Prove The Identity Sec X Csc 2x 2 Tanx Bartleby

What Is The Differentiation Of Sec Inverse Tan 2x Quora

4 Tan 2x 2 Sec 2x 1 0 X In

Sin2x Secx 1 Sec2x Sec2x 1 Brainly In

Incoming Term: tan^2x+1=sec^2x proof, tan^2(x) + 1 = sec^2(x) proof,